2023-01-11
Sub:

Moved to 연결사,connective.
하지만 2021-07-06부터 여기도 내용 적기로 함.

(2024-02-24) 저쪽에 통합하는게 나을 듯? 페이지명이 길어서... 굳이 자연어의 연결사들에 대한 내용을 내가 적을 필요는 없을 듯 한데.

2023-01-10
논리연산자,logical_operator와 완전히 같은 뜻인듯? chk

// 여기는 Namu: 명제 논리 참조함.

	항수		논	C	Cb	A
부정=negation	1	NOT	￢	!	~	-	P가 아니다
선언=논리합=disjunction	2	OR	∨	｜	｜｜	+	P또는 Q가 성립, 둘 다도 ok
배타적선언=배타논리합=exclusive_or	2	XOR	⊕		^		P또는 Q가 성립, 둘 중 하나만
연언=conjunction=논리곱	2	AND	∧	&&	&	·, ­×	P이고 Q
조건문=conditional	2		→				if P then Q
쌍조건문=biconditional	2		↔				오직 P인 경우에만 Q이다, iff

(맨 윗줄에 대해)
항수: 1이면 P, 2이면 P, Q를 가정
논: 논리 기호
C: C style? 기호
Cb: C bitwise operator 기호 (논리 기호는 아니지만 참고로)
A: 수식 유래 기호? .. arithmetical? (이 둘은 명칭 정확히 모르겠음)

disjunction에서, P와 Q를 선언지,disjunct라고 함. //(operator - operand 같은?)
conjunction에서, P와 Q는 연언지,conjunct.

Twins:
https://planetmath.org/logicalconnective
http://www.aistudy.com/logic/connective.htm
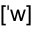logical_connective
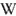Logical_connective
= https://en.wikipedia.org/wiki/Logical_connective
Connective
= https://mathworld.wolfram.com/Connective.html
tmp twins:
https://psychology.fandom.com/wiki/Logical_connective
https://en.wikibooks.org/wiki/Mathematical_Proof_and_the_Principles_of_Mathematics/Logic/Logical_connectives

Up: 논리상수,logical_constant