삼각함수,trigonometric_function
역삼각함수,inverse_trigonometric_function
쌍곡선함수,hyperbolic_function
역쌍곡선함수,inverse_hyperbolic_function
역삼각함수,inverse_trigonometric_function
쌍곡선함수,hyperbolic_function
역쌍곡선함수,inverse_hyperbolic_function
Sub:
삼각함수의_극한
삼각치환,trig_substitution
sine_function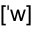 sine_function
sine_function  sine function
sine function
cosine_function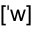 cosine_function
cosine_function  cosine function
cosine function
tangent_function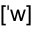 tangent_function
tangent_function  tangent function
tangent function
cotangent_function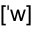 cotangent_function
cotangent_function  cotangent function
cotangent function
secant_function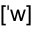 secant_function
secant_function  secant function
secant function
cosecant_function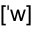 cosecant_function
cosecant_function  cosecant function
cosecant function
or
사인,sine
코사인,cosine
탄젠트,tangent ....?
rel sinusoid / sinusoidal_function 이었나? spell chk.
삼각치환,trig_substitution
| $\displaystyle \sin$ | $\displaystyle \csc$ |
| $\displaystyle \cos$ | $\displaystyle \sec$ |
| $\displaystyle \tan$ | $\displaystyle \cot$ |
sine_function
cosine_function
tangent_function
cotangent_function
secant_function
cosecant_function
or
사인,sine
코사인,cosine
탄젠트,tangent ....?
rel sinusoid / sinusoidal_function 이었나? spell chk.
1. 삼각함수 미분표 ¶
| (sin x)' = cos x | (csc x)' = - csc x cot x |
| (cos x)' = - sin x | (sec x)' = sec x tan x |
| (tan x)' = sec² x | (cot x)' = - csc² x |
| $\displaystyle \frac{d}{dx}\sin x$ | $\displaystyle \cos x$ | $\displaystyle \frac{d}{dx}\csc x$ | $\displaystyle -\csc x \cot x$ |
| $\displaystyle \frac{d}{dx}\cos x$ | $\displaystyle -\sin x$ | $\displaystyle \frac{d}{dx}\sec x$ | $\displaystyle \sec x \tan x $ |
| $\displaystyle \frac{d}{dx}\tan x$ | $\displaystyle \sec^2 x$ | $\displaystyle \frac{d}{dx}\cot x$ | $\displaystyle -\csc^2 x$ |
줄이 안맞아서 다른 방식으로.
$\displaystyle !mimetex $$\huge\begin{array}{|rl|rl|} \hline \frac{d}{dx}\sin x &= \cos x & \frac{d}{dx}\csc x &= -\csc x \cot x\\ \hdash \frac{d}{dx}\cos x &= -\sin x & \frac{d}{dx}\sec x &= \sec x \tan x \\ \hdash \frac{d}{dx}\tan x &= \sec^2 x & \frac{d}{dx}\cot x &= -\csc^2 x \\ \hline\end{array}$$
4. 삼각함수 극한 ¶
$\displaystyle \lim_{x\to0}\frac{\sin x}x=1$
이것은 보통 squeeze theorem으로 증명. 로피탈로도 가능.
넓이 부등식을...
$\displaystyle \frac12\cos x\sin x<\frac{x}2<\frac12\tan x$
$\displaystyle \cos x<\frac{x}{\sin x}<\frac{1}{\cos x}$
$\displaystyle \cos x<\frac{\sin x}{x}<\frac{1}{\cos x}$
$\displaystyle \lim_{x\to 0}\cos x<\lim_{x\to 0}\frac{\sin x}{x}<\lim_{x\to 0}\frac{1}{\cos x}$
이것은 보통 squeeze theorem으로 증명. 로피탈로도 가능.
넓이 부등식을...
$\displaystyle \frac12\cos x\sin x<\frac{x}2<\frac12\tan x$
$\displaystyle \cos x<\frac{x}{\sin x}<\frac{1}{\cos x}$
$\displaystyle \cos x<\frac{\sin x}{x}<\frac{1}{\cos x}$
$\displaystyle \lim_{x\to 0}\cos x<\lim_{x\to 0}\frac{\sin x}{x}<\lim_{x\to 0}\frac{1}{\cos x}$
$\displaystyle \lim_{x\to0}\frac{\cos x-1}{x}=0$
